Some of you may be know that, using a compass and unmarked
straightedge, it's impossible to trisect any given angle.
However, there are a number of ways to trisect any given angle
using a particular tool or object. One such object is a carpenter's
square.
A modern carpenter's square isn't really a square in the geometric
sense, but is rather a metal L with one arm that's a little
wider than the other. Carpenters use it for many different tasks
in building a house, and ultimately it contributes to the house
being "square" - meaning things are all at the correct angles.
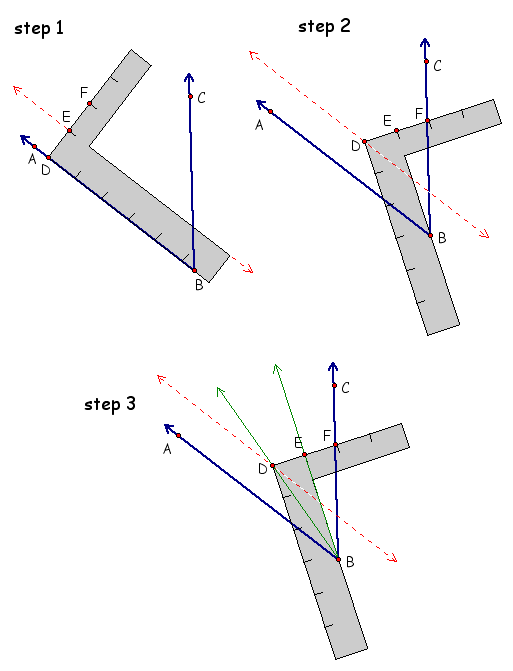
We're trying to trisect angle ABC. DE is equal to the width
of the wide arm and so is EF.
1.Use the wide arm of the square to draw a line parallel to
AB.
2.Lay the square so that one edge goes through B and the outer
corner, D, lies on the new line, and F lies on CB.
3.Draw rays BD and BE.
Are rays BD and BE really trisectors of angle ABC?